Does The Moon Change Its Position In The Sky Throughout The Year?

Not to be confused with Lunar orbit, the orbit of an object around the Moon.

The Moon's circuit effectually the Earth

Orbit of the Moon

Diagram of the Moon'southward orbit with respect to the Earth. While angles and relative sizes are to calibration, distances are not.

Property Value Semi-major axis [1] 384,748 km (239,071 mi)[2] Hateful distance [iii] 385,000 km (239,000 mi)[four] Inverse sine parallax [7] 384,400 km (238,900 mi) Perigee (i.due east., min. distance from Earth) 363,228.ix km (225,700.0 mi), avg. (356400 –370400  km) Apogee (i.e., max. altitude from World) 405,400 km (251,900 mi), avg. (404000 –406700  km) Mean eccentricity 0.0549006 (0.026–0.077)[five] Mean obliquity half dozen.687°[eight] Hateful inclination of orbit to ecliptic 5.15° (4.99–5.30)[v] of lunar equator to ecliptic 1.543° Menstruation of orbit effectually Globe (sidereal) 27.322 days orbit around Earth (synodic) 29.530 days precession of nodes 18.5996 years precession of line of apsides eight.8504 years

The Moon orbits Earth in the prograde direction and completes 1 revolution relative to the Vernal Equinox and the stars in almost 27.32 days (a tropical month and sidereal month) and 1 revolution relative to the Sun in about 29.53 days (a synodic month). Earth and the Moon orbit about their barycentre (common centre of mass), which lies about iv,670 km (2,900 mi) from Earth'due south center (about 73% of its radius), forming a satellite organization called the Earth–Moon system. On average, the distance to the Moon is about 385,000 km (239,000 mi) from Globe's center, which corresponds to nigh threescore Earth radii or 1.282 low-cal-seconds.

With a mean orbital velocity of i.022 km/due south (0.635 miles/s),^[nine] the Moon covers a distance approximately its diameter, or about half a degree on the celestial sphere, each hour. The Moon differs from most satellites of other planets in that its orbit is close to the ecliptic airplane instead of to its principal's (in this case, Earth's) equatorial plane. The Moon'due south orbital airplane is inclined by virtually five.i° with respect to the ecliptic aeroplane, whereas the Moon's equatorial airplane is tilted by only 1.5°.

Properties [edit]

The backdrop of the orbit described in this section are approximations. The Moon'south orbit around Earth has many variations (perturbations) due to the gravitational attraction of the Lord's day and planets, the study of which (lunar theory) has a long history.^[10]

Moon'due south orbit and sizes of Globe and Moon to calibration.

Elliptic shape [edit]

The orbit of the Moon is a nearly round ellipse about the Globe (the semimajor and semiminor axes are 384,400 km and 383,800 km, respectively: a difference of only 0.16%). The equation of the ellipse yields an eccentricity of 0.0549 and perigee and apogee distances of 362,600 km and 405,400 km respectively (a divergence of 12%).

Since nearer objects announced larger, the Moon'due south apparent size changes every bit it moves toward and away from an observer on Earth. An event referred to as a 'supermoon' occurs when the full Moon is at its closest to Earth (perigee). The largest possible credible diameter of the Moon is the same 12% larger (as perigee versus apogee distances) than the smallest; the credible area is 25% more and so is the corporeality of light it reflects toward Earth.

The variance in the Moon'south orbital distance corresponds with changes in its tangential and angular speeds, equally stated in Kepler's second constabulary. The hateful angular motion relative to an imaginary observer at the Earth–Moon barycentre is 13.176° per day to the east (J2000.0 epoch).

Minimum, mean and maximum distances of the Moon from Globe with its angular diameter as seen from World's surface, to scale

Elongation [edit]

The Moon'due south elongation is its athwart distance east of the Sunday at any time. At new moon, it is zip and the Moon is said to be in conjunction. At total moon, the elongation is 180° and it is said to exist in opposition. In both cases, the Moon is in syzygy, that is, the Sun, Moon and Earth are about aligned. When elongation is either xc° or 270°, the Moon is said to exist in quadrature.

Precession [edit]

Apsidal precession—The major axis of Moon'due south elliptical orbit rotates past one complete revolution in one case every eight.85 years in the same management equally the Moon's rotation itself. This prototype looks upwards depicting Globe's geographic southward pole and the elliptical shape of the Moon'south orbit (vastly exaggerated from its almost circular shape to make the precession evident) is rotating from white to greyer orbits.

Animation of Moon orbit around Earth
 Moon·

 Globe
Summit: polar view; bottom: equatorial view

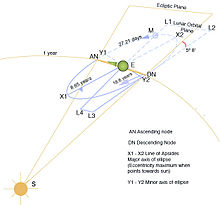
Earth's lunar orbit perturbations

The orientation of the orbit is not stock-still in space merely rotates over time. This orbital precession is called apsidal precession and is the rotation of the Moon's orbit inside the orbital plane, i.e. the axes of the ellipse alter direction. The lunar orbit's major axis – the longest diameter of the orbit, joining its nearest and uttermost points, the perigee and apogee, respectively – makes 1 consummate revolution every 8.85 Earth years, or 3,232.6054 days, as information technology rotates slowly in the same direction equally the Moon itself (direct motion) - meaning precesses east by 360°. The Moon's apsidal precession is distinct from the nodal precession of its orbital plane and centric precession of the moon itself.

Inclination [edit]

The mean inclination of the lunar orbit to the ecliptic plane is 5.145°. Theoretical considerations show that the present inclination relative to the ecliptic plane arose past tidal evolution from an earlier most-Earth orbit with a fairly constant inclination relative to World'south equator.^[11] It would require an inclination of this earlier orbit of about 10° to the equator to produce a present inclination of 5° to the ecliptic. It is thought that originally the inclination to the equator was near zero, simply it could have been increased to 10° through the influence of planetesimals passing near the Moon while falling to the Earth.^[12] If this had non happened, the Moon would now lie much closer to the ecliptic and eclipses would be much more frequent.^[thirteen]

The rotational axis of the Moon is not perpendicular to its orbital plane, so the lunar equator is not in the plane of its orbit, but is inclined to it by a constant value of 6.688° (this is the obliquity). As was discovered by Jacques Cassini in 1722, the rotational axis of the Moon precesses with the same rate as its orbital airplane, but is 180° out of stage (see Cassini'south Laws). Therefore, the angle betwixt the ecliptic and the lunar equator is ever 1.543°, even though the rotational axis of the Moon is not fixed with respect to the stars.^[14]

Nodes [edit]

The nodes are points at which the Moon's orbit crosses the ecliptic. The Moon crosses the same node every 27.2122 days, an interval called the draconic calendar month or draconitic month. The line of nodes, the intersection between the two corresponding planes, has a retrograde motion: for an observer on Earth, it rotates westward along the ecliptic with a flow of 18.6 years or xix.3549° per twelvemonth. When viewed from the celestial north, the nodes movement clockwise around Earth, opposite to World'due south ain spin and its revolution effectually the Lord's day. An Eclipse of the Moon or Sun can occur when the nodes align with the Sun, roughly every 173.3 days. Lunar orbit inclination also determines eclipses; shadows cross when nodes coincide with full and new moon when the Dominicus, Globe, and Moon marshal in three dimensions.

In effect, this means that the "tropical year" on the Moon is only 347 days long. This is called the draconic yr or eclipse yr. The "seasons" on the Moon fit into this menstruum. For about one-half of this draconic year, the Sun is north of the lunar equator (but at most i.543°), and for the other half, it is south of the lunar equator. Evidently, the outcome of these seasons is minor compared to the deviation between lunar night and lunar day. At the lunar poles, instead of usual lunar days and nights of about 15 Earth days, the Sun will be "up" for 173 days equally it will be "downward"; polar sunrise and sunset takes xviii days each year. "Up" here means that the center of the Sunday is in a higher place the horizon.^[xv] Lunar polar sunrises and sunsets occur around the fourth dimension of eclipses (solar or lunar). For example, at the Solar eclipse of March 9, 2016, the Moon was virtually its descending node, and the Dominicus was near the point in the sky where the equator of the Moon crosses the ecliptic. When the Sun reaches that point, the eye of the Sun sets at the lunar north pole and rises at the lunar south pole.

The solar eclipse of September 1 of the same year, the Moon was near its ascending node, and the Sun was near the point in the sky where the equator of the Moon crosses the ecliptic. When the Sun reaches that indicate, the center of the Sun rises at the lunar north pole and sets at the lunar south pole.

Inclination to the equator and lunar standstill [edit]

Every 18.6 years, the bending between the Moon'southward orbit and Earth's equator reaches a maximum of 28°36′, the sum of Earth'due south equatorial tilt (23°27′) and the Moon'due south orbital inclination (5°09′) to the ecliptic. This is called major lunar standstill. Around this time, the Moon'southward declination volition vary from −28°36′ to +28°36′. Conversely, 9.3 years subsequently, the angle between the Moon'southward orbit and Earth's equator reaches its minimum of 18°20′. This is chosen a small-scale lunar standstill. The last lunar standstill was a pocket-sized standstill in October 2015. At that time the descending node was lined up with the equinox (the point in the heaven having right ascension aught and declination zero). The nodes are moving w by most 19° per twelvemonth. The Sun crosses a given node nigh 20 days before each twelvemonth.

When the inclination of the Moon'due south orbit to the Earth'southward equator is at its minimum of xviii°20′, the centre of the Moon's deejay will be in a higher place the horizon every solar day from latitudes less than 70°43' (90° − 18°20' – 57' parallax) north or south. When the inclination is at its maximum of 28°36', the centre of the Moon's disk will be above the horizon every day only from latitudes less than lx°27' (90° − 28°36' – 57' parallax) n or southward.

At higher latitudes, at that place volition be a period of at to the lowest degree one day each calendar month when the Moon does not rise, only there volition also exist a period of at least one twenty-four hour period each month when the Moon does non set. This is similar to the seasonal behaviour of the Sun, but with a period of 27.2 days instead of 365 days. Notation that a indicate on the Moon can actually be visible when it is about 34 arc minutes below the horizon, due to atmospheric refraction.

Because of the inclination of the Moon'south orbit with respect to the Earth'due south equator, the Moon is to a higher place the horizon at the N and Southward Pole for nearly 2 weeks every month, even though the Sun is below the horizon for six months at a time. The period from moonrise to moonrise at the poles is a tropical month, about 27.3 days, quite close to the sidereal flow. When the Sun is the furthest beneath the horizon (winter solstice), the Moon volition be full when it is at its highest point. When the Moon is in Gemini it will be above the horizon at the North Pole, and when it is in Sagittarius information technology will be upwardly at the South Pole.

The Moon'due south light is used by zooplankton in the Chill when the Lord's day is below the horizon for months^[16] and must take been helpful to the animals that lived in Arctic and Antarctic regions when the climate was warmer.

Scale model [edit]

• 

  Scale model of the World–Moon system: Sizes and distances are to scale. Information technology represents the hateful distance of the orbit and the mean radii of both bodies.

History of observations and measurements [edit]

The credible trajectory of the Moon in the sky seen from World each night is like a broad ellipse, although the path depends on the fourth dimension of the year and latitude.

About 1000 BC, the Babylonians were the beginning human civilization known to accept kept a consistent record of lunar observations. Clay tablets from that period, which have been found over the territory of present-day Iraq, are inscribed with cuneiform writing recording the times and dates of moonrises and moonsets, the stars that the Moon passed close by, and the fourth dimension differences between rising and setting of both the Sun and the Moon around the time of the total moon. Babylonian astronomy discovered the three chief periods of the Moon's motion and used data analysis to build lunar calendars that extended well into the future.^[10] This use of detailed, systematic observations to brand predictions based on experimental data may be classified as the first scientific written report in homo history. All the same, the Babylonians seem to take lacked any geometrical or physical interpretation of their data, and they could not predict time to come lunar eclipses (although "warnings" were issued before probable eclipse times).

Ancient Greek astronomers were the first to introduce and clarify mathematical models of the movement of objects in the sky. Ptolemy described lunar movement past using a well-defined geometric model of epicycles and evection.^[10]

Sir Isaac Newton was the beginning to develop a complete theory of motility, mechanics. The observations of the lunar motion were the main examination of his theory.^[ten]

Lunar periods [edit]

Name	Value (days)	Definition
Sidereal month	27.321662	with respect to the distant stars (xiii.36874634 passes per solar orbit)
Synodic month	29.530589	with respect to the Dominicus (phases of the Moon, 12.36874634 passes per solar orbit)
Tropical month	27.321582	with respect to the vernal point (precesses in ~26,000 years)
Anomalistic month	27.554550	with respect to the perigee (precesses in 3232.6054 days = 8.850578 years)
Draconic calendar month	27.212221	with respect to the ascending node (precesses in 6793.4765 days = 18.5996 years)

There are several unlike periods associated with the lunar orbit.^[17] The sidereal calendar month is the time it takes to brand i complete orbit around Earth with respect to the fixed stars. It is about 27.32 days. The synodic month is the time it takes the Moon to reach the aforementioned visual phase. This varies notably throughout the yr,^[18] but averages around 29.53 days. The synodic catamenia is longer than the sidereal period because the Earth–Moon system moves in its orbit around the Sunday during each sidereal month, hence a longer period is required to accomplish a similar alignment of Earth, the Sun, and the Moon. The anomalistic month is the fourth dimension between perigees and is about 27.55 days. The Earth–Moon separation determines the force of the lunar tide raising force.

The draconic month is the time from ascending node to ascending node. The fourth dimension between 2 successive passes of the aforementioned ecliptic longitude is called the tropical month. The latter periods are slightly different from the sidereal month.

The boilerplate length of a month (a 12th of a yr) is about 30.4 days. This is non a lunar period, though the month is historically related to the visible lunar phase.

Tidal evolution [edit]

The gravitational attraction that the Moon exerts on Globe is the cause of tides in both the ocean and the solid Earth; the Sun has a smaller tidal influence. The solid Earth responds quickly to any change in the tidal forcing, the distortion taking the form of an ellipsoid with the high points roughly beneath the Moon and on the opposite side of World. This is a outcome of the high speed of seismic waves within the solid Earth.

However the speed of seismic waves is not infinite and, together with the effect of free energy loss within the Globe, this causes a slight filibuster between the passage of the maximum forcing due to the Moon across and the maximum Globe tide. Equally the World rotates faster than the Moon travels effectually its orbit, this small angle produces a gravitational torque which slows the Earth and accelerates the Moon in its orbit.

In the case of the body of water tides, the speed of tidal waves in the ocean^[19] is far slower than the speed of the Moon'due south tidal forcing. Every bit a result, the sea is never in near equilibrium with the tidal forcing. Instead, the forcing generates the long ocean waves which propagate around the ocean basins until somewhen losing their energy through turbulence, either in the deep sea or on shallow continental shelves.

Although the ocean's response is the more complex of the ii, information technology is possible to split the ocean tides into a small ellipsoid term which affects the Moon plus a second term which has no result. The ocean'due south ellipsoid term also slows the Earth and accelerates the Moon, but because the sea dissipates so much tidal energy, the present ocean tides have an order of magnitude greater effect than the solid World tides.

Because of the tidal torque, acquired by the ellipsoids, some of Earth's angular (or rotational) momentum is gradually being transferred to the rotation of the Earth–Moon pair around their common centre of mass, called the barycentre. Run across tidal dispatch for a more than detailed description.

This slightly greater orbital athwart momentum causes the World–Moon distance to increase at approximately 38 millimetres per yr.^[xx] Conservation of angular momentum means that Earth's centric rotation is gradually slowing, and because of this its day lengthens by approximately 24 microseconds every year (excluding glacial rebound). Both figures are valid only for the current configuration of the continents. Tidal rhythmites from 620 million years ago testify that, over hundreds of millions of years, the Moon receded at an average charge per unit of 22 mm (0.87 in) per year (2200 km or 0.56% or the Earth-moon distance per hundred one thousand thousand years) and the twenty-four hour period lengthened at an average rate of 12 microseconds per yr (or 20 minutes per hundred one thousand thousand years), both virtually half of their current values.

The nowadays loftier rate may be due to near resonance between natural ocean frequencies and tidal frequencies.^[21] Another explanation is that in the by the Globe rotated much faster, a day possibly lasting only 9 hours on the early Earth. The resulting tidal waves in the ocean would have then been much shorter and information technology would have been more difficult for the long wavelength tidal forcing to excite the brusk wavelength tides.^[22]

The Moon is gradually receding from Globe into a higher orbit, and calculations advise that this would continue for about 50 billion years.^{[23] [24]} By that time, Globe and the Moon would be in a mutual spin–orbit resonance or tidal locking, in which the Moon will orbit Earth in about 47 days (currently 27 days), and both the Moon and Earth would rotate around their axes in the aforementioned fourth dimension, ever facing each other with the same side. This has already happened to the Moon—the same side always faces Earth—and is besides slowly happening to the Earth. However, the slowdown of Earth's rotation is not occurring fast enough for the rotation to lengthen to a month before other effects alter the situation: approximately 2.three billion years from at present, the increase of the Sunday'south radiation will accept caused Globe'due south oceans to evaporate,^[25] removing the bulk of the tidal friction and dispatch.

Libration [edit]

Animation of the Moon as information technology cycles through its phases. The apparent wobbling of the Moon is known as libration.

The Moon is in synchronous rotation, meaning that information technology keeps the aforementioned face up toward Globe at all times. This synchronous rotation is only true on average because the Moon's orbit has a definite eccentricity. As a result, the angular velocity of the Moon varies as information technology orbits Earth and hence is non ever equal to the Moon'south rotational velocity which is more than constant. When the Moon is at its perigee, its orbital motion is faster than its rotation. At that fourth dimension the Moon is a bit ahead in its orbit with respect to its rotation about its axis, and this creates a perspective upshot which allows us to see up to 8 degrees of longitude of its eastern (right) far side. Conversely, when the Moon reaches its apogee, its orbital motility is slower than its rotation, revealing viii degrees of longitude of its western (left) far side. This is referred to as optical libration in longitude.

The Moon's axis of rotation is inclined past in full 6.7° relative to the normal to the plane of the ecliptic. This leads to a similar perspective result in the due north–south direction that is referred to equally optical libration in latitude, which allows one to encounter about seven° of latitude across the pole on the far side. Finally, because the Moon is merely about threescore Globe radii away from Earth's heart of mass, an observer at the equator who observes the Moon throughout the dark moves laterally by i Earth diameter. This gives rising to a diurnal libration, which allows one to view an additional i degree'due south worth of lunar longitude. For the aforementioned reason, observers at both of Earth's geographical poles would be able to run into 1 additional degree's worth of libration in latitude.

Besides these "optical librations" caused past the alter in perspective for an observer on Globe, there are likewise "physical librations" which are actual nutations of the direction of the pole of rotation of the Moon in space: but these are very modest.

Path of Earth and Moon around Sunday [edit]

Department of Globe's and Moon'south trajectories around the Sun^[26]

When viewed from the north celestial pole (i.e., from the estimate management of the star Polaris) the Moon orbits Earth anticlockwise and Earth orbits the Sun anticlockwise, and the Moon and World rotate on their own axes anticlockwise.

The right-manus rule can be used to point the direction of the angular velocity. If the thumb of the right hand points to the north celestial pole, its fingers curl in the direction that the Moon orbits Earth, Earth orbits the Sun, and the Moon and Globe rotate on their own axes.

In representations of the Solar System, it is mutual to draw the trajectory of Earth from the point of view of the Sun, and the trajectory of the Moon from the point of view of Earth. This could give the impression that the Moon orbits Globe in such a manner that sometimes information technology goes backwards when viewed from the Sun'due south perspective. All the same, considering the orbital velocity of the Moon effectually Earth (1 km/s) is small compared to the orbital velocity of Earth well-nigh the Sun (30 km/s), this never happens. There are no rearward loops in the Moon'south solar orbit.

Considering the World–Moon arrangement as a binary planet, its centre of gravity is within Earth, nigh 4,671 km (two,902 mi)^[27] or 73.iii% of the Earth's radius from the heart of the World. This eye of gravity remains on the line between the centres of the Earth and Moon as the Earth completes its diurnal rotation. The path of the World–Moon system in its solar orbit is defined equally the movement of this mutual heart of gravity around the Sun. Consequently, World's middle veers inside and outside the solar orbital path during each synodic month as the Moon moves in its orbit effectually the common centre of gravity.^[28]

The Lord's day's gravitational effect on the Moon is more than twice that of Earth'southward on the Moon; consequently, the Moon's trajectory is always convex^{[28] [29]} (as seen when looking Sunward at the entire Sun–World–Moon organisation from a not bad distance exterior Earth–Moon solar orbit), and is nowhere concave (from the aforementioned perspective) or looped.^{[26] [28] [30]} That is, the region enclosed by the Moon'southward orbit of the Sun is a convex set.

See besides [edit]

• Ernest William Brownish
• Double planet
• List of orbits
• ELP2000
• Ephemeris
• Jet Propulsion Laboratory Development Ephemeris
• Lunar altitude (astronomy)
• Lunar Laser Ranging Experiment
• Lunar phase
• Lunar theory
• Milankovitch cycles
• Orbital elements
• Supermoon

References [edit]

1. ^ The geometric mean distance in the orbit (of ELP) which is the semimajor axis of the Moon's elliptical orbit via Kepler's laws
2. ^ Grand. Chapront-Touzé; J. Chapront (1983). "The lunar ephemeris ELP-2000". Astronomy & Astrophysics. 124: 54. Bibcode:1983A&A...124...50C.
3. ^ The abiding in the ELP expressions for the distance, which is the mean distance averaged over time
4. ^ Yard. Chapront-Touzé; J. Chapront (1988). "ELP2000-85: a semi-analytical lunar ephemeris adequate for historical times". Astronomy & Astrophysics. 190: 351. Bibcode:1988A&A...190..342C.
5. ^ ^{a b c} Meeus, Jean (1997), Mathematical Astronomy Morsels, Richmond, VA: Willmann-Bell, pp. 11–12, 22–23, ISBN0-943396-51-4
6. ^ Seidelmann, P. Kenneth, ed. (1992), Explanatory Supplement to the Astronomical Almanac, University Science Books, pp. 696, 701, ISBN0-935702-68-7
7. ^ The inverse sine parallax ɑ / sin π is traditionally the Moon's mean distance from Earth (center to center), where ɑ is Globe's equatorial radius, and π is the Moon's parallax between the ends of ɑ.^[5] Iii of the IAU 1976 Astronomical Constants were "mean distance of Moon from Earth" 384,400km, "equatorial horizontal parallax at mean distance" 3422.608″, and "equatorial radius for Earth" 6,378.fourteenkm.^{[half dozen]}
8. ^ Lang, Kenneth R. (2011), The Cambridge Guide to the Solar System, 2nd ed., Cambridge University Press.
9. ^ "Moon Fact Canvass". NASA. Retrieved 2014-01-08 .
10. ^ ^{a b c d} Martin C. Gutzwiller (1998). "Moon-Earth-Lord's day: The oldest iii-body problem". Reviews of Modern Physics. lxx (2): 589–639. Bibcode:1998RvMP...seventy..589G. doi:10.1103/RevModPhys.seventy.589.
11. ^ Peter Goldreich (Nov 1966). "History of the Lunar Orbit". Reviews of Geophysics. 4 (4): 411. Bibcode:1966RvGSP...iv..411G. doi:10.1029/RG004i004p00411. Jihad Touma & Jack Wisdom (Nov 1994). "Evolution of the Earth-Moon system". The Astronomical Periodical. 108: 1943. Bibcode:1994AJ....108.1943T. doi:10.1086/117209.
12. ^ Kaveh Pahlevan & Alessandro Morbidelli (Nov 26, 2015). "Collisionless encounters and the origin of the lunar inclination". Nature. 527 (7579): 492–494. arXiv:1603.06515. Bibcode:2015Natur.527..492P. doi:10.1038/nature16137. PMID 26607544. S2CID 4456736.
13. ^ Jacob Aron (November 28, 2015). "Flying gold knocked the moon off course and ruined eclipses". New Scientist.
14. ^ "View of the Moon". U. of Arkansas at Niggling Rock. Retrieved May 9, 2016.
15. ^ Calculated from arcsin(0.25°/i.543°)/90° times 173 days, since the angular radius of the Sun is about 0.25°.
16. ^ "Moonlight helps plankton escape predators during Arctic winters". New Scientist. January sixteen, 2016.
17. ^ The periods are calculated from orbital elements, using the rate of change of quantities at the instant J2000. The J2000 rate of modify equals the coefficient of the starting time-degree term of VSOP polynomials. In the original VSOP87 elements, the units are arcseconds(") and Julian centuries. There are 1,296,000" in a circle, 36525 days in a Julian century. The sidereal month is the time of a revolution of longitude λ with respect to the fixed J2000 equinox. VSOP87 gives 1732559343.7306" or 1336.8513455 revolutions in 36525 days–27.321661547 days per revolution. The tropical month is similar, but the longitude for the equinox of appointment is used. For the anomalistic yr, the mean anomaly (λ-ω) is used (equinox does non matter). For the draconic month, (λ-Ω) is used. For the synodic month, the sidereal flow of the mean Sun (or Earth) and the Moon. The menses would be ane/(one/one thousand-ane/e). VSOP elements from Simon, J.Fifty.; Bretagnon, P.; Chapront, J.; Chapront-Touzé, M.; Francou, 1000.; Laskar, J. (February 1994). "Numerical expressions for precession formulae and hateful elements for the Moon and planets". Astronomy and Astrophysics. 282 (two): 669. Bibcode:1994A&A...282..663S.
18. ^ Jean Meeus, Astronomical Algorithms (Richmond, VA: Willmann-Bell, 1998) p 354. From 1900–2100, the shortest time from one new moon to the side by side is 29 days, 6 hours, and 35 min, and the longest 29 days, xix hours, and 55 min.
19. ^ J.B. Zirkir (2013). The Science of Ocean Waves. Johns Hopkins Academy Printing. p. 264. ISBN9781421410784.
20. ^ Williams, James G.; Boggs, Dale H. (2016). "Secular tidal changes in lunar orbit and World rotation". Celestial Mechanics and Dynamical Astronomy. 126 (one): 89–129. Bibcode:2016CeMDA.126...89W. doi:10.1007/s10569-016-9702-3. ISSN 0923-2958. S2CID 124256137.
21. ^ Williams, George E. (2000). "Geological constraints on the Precambrian history of Earth'due south rotation and the Moon's orbit". Reviews of Geophysics. 38 (one): 37–60. Bibcode:2000RvGeo..38...37W. doi:ten.1029/1999RG900016.
22. ^ Webb, David J. (1982). "Tides and the evolution of the Earth-Moon organization". Geophysical Journal of the Royal Astronomical Gild. seventy (1): 261–271. Bibcode:1982GeoJ...seventy..261W. doi:10.1111/j.1365-246X.1982.tb06404.x.
23. ^ C.D. Murray; South.F. Dermott (1999). Solar System Dynamics. Cambridge University Press. p. 184.
24. ^ Dickinson, Terence (1993). From the Big Bang to Planet 10. Camden E, Ontario: Camden House. pp. 79–81. ISBN0-921820-71-2.
25. ^ Caltech Scientists Predict Greater Longevity for Planets with Life Archived 2012-03-thirty at the Wayback Machine
26. ^ ^{a b} The reference by H. 50. Vacher (2001) (details separately cited in this list) describes this as 'convex outward', whereas older references such as "The Moon's Orbit Around the Sun, Turner, A. B. Journal of the Royal Astronomical Order of Canada, Vol. six, p. 117, 1912JRASC...vi..117T"; and "H Godfray, Elementary Treatise on the Lunar Theory" draw the aforementioned geometry by the words concave to the sun.
27. ^ Seidelmann, P. Kenneth, ed. (1992), Explanatory Supplement to the Astronomical Almanac, University Science Books, p. 701, ISBN0-935702-68-7
28. ^ ^{a b c} Aslaksen, Helmer (2010). "The Orbit of the Moon effectually the Dominicus is Convex!". Retrieved 2006-04-21 .
29. ^ The Moon Ever Veers Toward the Sun at MathPages
30. ^ Vacher, H.50. (November 2001). "Computational Geology eighteen – Definition and the Concept of Set" (PDF). Periodical of Geoscience Education. 49 (5): 470–479. Retrieved 2006-04-21 .

External links [edit]

• View of the Moon Good diagrams of Moon, Earth, tilts of orbits and axes, courtesy of U. of Arkansas

Source: https://en.wikipedia.org/wiki/Orbit_of_the_Moon

Posted by: birchdelitth60.blogspot.com

Share this post